

AES INDUSTRIES LLC
Introduces . . . an engineered solution . . . for Automotive Engine Efficiency . .
. . . Simplified CVVT (Continuous Variable Valve Timing)
Your Subtitle text
The Problem & Solution
Preface;
To impart phase shifting CVVT between the two pulleys, slack is introduced to the geometry with a longer belt / chain length that is manipulated between two idlers.
The examples below depicts one half view of two timing pulleys (drive and driven) with a timing belt between. Upper portion of picture represents each belt position length shown in a straight linear line.
____________
To impart phase shifting CVVT between the two pulleys, slack is introduced to the geometry with a longer belt / chain length that is manipulated between two idlers.
The examples below depicts one half view of two timing pulleys (drive and driven) with a timing belt between. Upper portion of picture represents each belt position length shown in a straight linear line.
____________
The Problem (101); Non-Linear length change for the belt path!
As observed in many USA patented geometries.
[Straight move geometry, on-center, 1:1 ratio]
In picture below, advance slides to see relative length changes;
[Idler positions and line spacing are equal units]
1) Belt length between the two pulleys (shortest)
2 - 5) Idler pulley engaged with the belt in at least a first - fourth positions
6) Shows parabolic relationship with each position as it relates to length changes from each position from first to fourth.
As observed in many USA patented geometries.
[Straight move geometry, on-center, 1:1 ratio]
In picture below, advance slides to see relative length changes;
[Idler positions and line spacing are equal units]
1) Belt length between the two pulleys (shortest)
2 - 5) Idler pulley engaged with the belt in at least a first - fourth positions
6) Shows parabolic relationship with each position as it relates to length changes from each position from first to fourth.
Conclusion (101);
Because the belt cannot change its length, the geometry must maintain a consistent path length as the slack is manipulated for phase shifting. This is an obvious requirement of the geometry, however the geometry arrangement above fails to provide a consistent path length for the belt. This is demonstrated in slide #6 showing a curved graphed relationship to each length change.
_______________
Because the belt cannot change its length, the geometry must maintain a consistent path length as the slack is manipulated for phase shifting. This is an obvious requirement of the geometry, however the geometry arrangement above fails to provide a consistent path length for the belt. This is demonstrated in slide #6 showing a curved graphed relationship to each length change.
_______________
The Problem (102); Non-Linear length change for the belt path!
[Straight move geometry, off-center, 1:1 ratio]
Scroll through pictures below to see relative length changes;
1) Belt length between the two pulleys [shortest]
2 - 4) Idler pulley engaged with the belt in at least a first - third positions
5) Shows parabolic relationship with each position as it relates to length changes from each position from first to third.
[Straight move geometry, off-center, 1:1 ratio]
Scroll through pictures below to see relative length changes;
1) Belt length between the two pulleys [shortest]
2 - 4) Idler pulley engaged with the belt in at least a first - third positions
5) Shows parabolic relationship with each position as it relates to length changes from each position from first to third.
Conclusion (102);
Again, the geometry arrangement above fails to provide a consistent path length for the belt. This is demonstrated in slide #5 showing a curved graphed relationship to each length change.
_______________
Again, the geometry arrangement above fails to provide a consistent path length for the belt. This is demonstrated in slide #5 showing a curved graphed relationship to each length change.
_______________
The Problem (103); Non-Linear length change for the belt path! Observed Patent 3,496,918 "Finlay" 02.24.1970
[Straight move geometry, center, 2:1 ratio]
Scroll through pictures below to see relative length changes;
1) Belt length between the two pulleys [shortest]
2 - 5) Idler pulley engaged with the belt in at least a first - fourth positions
6) Shows parabolic relationship with each position as it relates to length changes from each position from first to fourth.
[Straight move geometry, center, 2:1 ratio]
Scroll through pictures below to see relative length changes;
1) Belt length between the two pulleys [shortest]
2 - 5) Idler pulley engaged with the belt in at least a first - fourth positions
6) Shows parabolic relationship with each position as it relates to length changes from each position from first to fourth.
Conclusion (103);
Again, the geometry arrangement above fails to provide a consistent path length for the belt. This is demonstrated in slide #6 showing a curved graphed relationship to each length change. The geometry above is therefore not valid and never gained acceptance due to increasing tension during manipulation with inconsistent timing losses. Many patents are very similar to this one and each one suffering from the same fate.
_____________
Again, the geometry arrangement above fails to provide a consistent path length for the belt. This is demonstrated in slide #6 showing a curved graphed relationship to each length change. The geometry above is therefore not valid and never gained acceptance due to increasing tension during manipulation with inconsistent timing losses. Many patents are very similar to this one and each one suffering from the same fate.
_____________
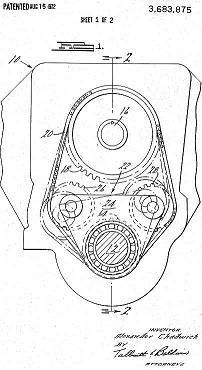
The Problem (104); Non-Linear length change for the belt path! Observed Patent 3,683,875 "Chadwick" 08.15.1972
[Crankshaft centered geometry, 2:1 ratio]
Scroll through pictures below to see relative length changes;
1) Belt length between the two pulleys [shortest]
2 - 5) Idler pulley engaged with the belt in at least a first - fourth positions
6) Shows parabolic relationship with each position as it relates to length changes from each position from first to fourth.
[Crankshaft centered geometry, 2:1 ratio]
Scroll through pictures below to see relative length changes;
1) Belt length between the two pulleys [shortest]
2 - 5) Idler pulley engaged with the belt in at least a first - fourth positions
6) Shows parabolic relationship with each position as it relates to length changes from each position from first to fourth.
Conclusion (104);
Again, the geometry arrangement above fails to provide a consistent path length for the belt. This is demonstrated in slide #6 showing a curved graphed relationship to each length change. This is why simple patented designs such as the Finlay and Chadwick were never utilized and the industry resorted to much more expensive alternatives pursuing the value added CVVT.
_____________
Again, the geometry arrangement above fails to provide a consistent path length for the belt. This is demonstrated in slide #6 showing a curved graphed relationship to each length change. This is why simple patented designs such as the Finlay and Chadwick were never utilized and the industry resorted to much more expensive alternatives pursuing the value added CVVT.
_____________
The Solution! Linear length change for the belt path!
Observed Patent 7,866,292 "LaBere" 01.11.2011
[Algorithm defined geometry, 2:1 ratio]
Scroll through pictures below to see relative length changes;
1) Belt length between the two pulleys [shortest]
2 - 5) Idler pulley engaged with the belt in at least a first - fourth positions
6) Shows parabolic relationship with each position as it relates to length changes from each position from first to fourth.
Observed Patent 7,866,292 "LaBere" 01.11.2011
[Algorithm defined geometry, 2:1 ratio]
Scroll through pictures below to see relative length changes;
1) Belt length between the two pulleys [shortest]
2 - 5) Idler pulley engaged with the belt in at least a first - fourth positions
6) Shows parabolic relationship with each position as it relates to length changes from each position from first to fourth.
Conclusion;
The geometry arrangement above provides a consistent path length for the belt. This is demonstrated in slide #6 showing a straight line graphed relationship to each length change. This simple design can now be utilized greatly reducing costs and providing a path to all existing basic cam driven valve timing engines. Even thirsty 1970's muscle cars can benefit!
_____________
The geometry arrangement above provides a consistent path length for the belt. This is demonstrated in slide #6 showing a straight line graphed relationship to each length change. This simple design can now be utilized greatly reducing costs and providing a path to all existing basic cam driven valve timing engines. Even thirsty 1970's muscle cars can benefit!
_____________
References:
all ^ www.USPTO.gov United States Patent and Trademark Office; Retrieved 2010-10-05; 2011-01-11
all ^ www.USPTO.gov United States Patent and Trademark Office; Retrieved 2010-10-05; 2011-01-11
